OS-V: 1010 Complex Eigenvalue Analysis of Rotor Bearing System
Rotor Bearing system is an excellent example of rotating machines used in mechanical engineering applications.
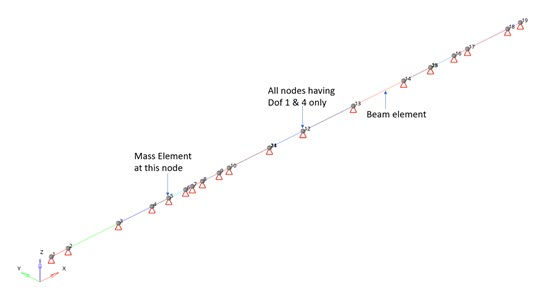
Figure 1. 1D Rotor Model
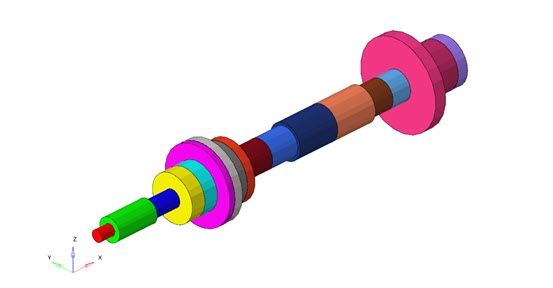
Figure 2. 3D Representation of Beams
Model Files
Benchmark Model
The finite element model, as shown in Figure 1 is constrained at all the nodes. Only DOF 1 and 4 are allowed on all the nodes. The model is meshed with beam elements of different sections (Figure 2). Mass is attached at node 5. An isotropic system is assumed.
Material
- Property
- Value
- Young's modulus
- 207.8 GN/m2
- Density
- 7806 kg/m3
- k22 = k33
- = 3.503 x e7 N/m
- k23 = k32
- = -8.756 N/m
- DMIG
- The stiffness matrix of the bearing is defined directly in the model as multiple column entries using K2GG.
- GENEL
- A file (.inc) which contains the details of bearing stiffness is imported in the model.
The problem has been solved for Complex Eigenvalue Analysis (ASYNC).
Compare the whirl speeds at spin speed being 100,000 RPM.
Results
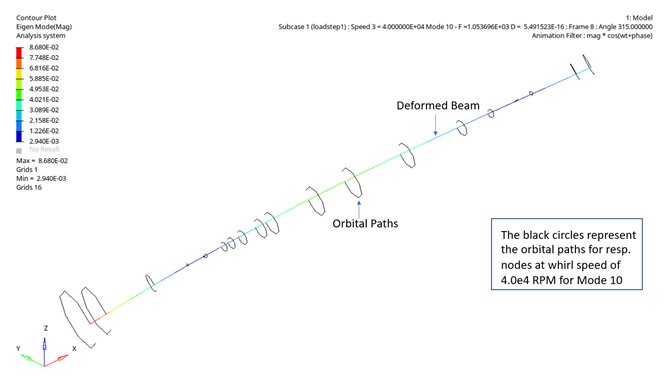
Figure 3. Eigen Mode Contour Plot for Spin Speed of 4.0e-4 RPM and 10th Mode
- Nomenclature
- Whirl Speed
- The damped natural frequency of the rotor.
- Backward Whirl (BW) and Forward Whirl (FW)
- At zero shaft speed, the forward and backward frequencies are identical (repeated eigenvalues). As speed increases, each vibration mode is split into two modes, known as forward and backward precision modes, due to gyroscopic effect.