Post-processing quantities: rotation motion

Introduction

This paragraph deals with physical quantities computed for rotating motion .

Kinematic variables

The list of kinematic variables used for solving and the names of the corresponding Flux parameters are given in the table below; in this table MS indicates the concerned mechanical set.

Kinematic variable	Name	Unit
Angular position:	AngPos(MS)	degree
Angular speed:	AngSpeed(MS)	degree/s
Angular acceleration:	AngAcc(MS)	degree/s²

Physical quantities

The list of physical quantities computed in the post-processing module and the names of the corresponding Flux parameters are given in the table below; in this table MS indicates the concerned mechanical set.

Torque	Name	Unit	Explanation
Electromagnetic torque:	TorqueElecMag(MS)	N.m
Internal system
Friction torque:	TorqueFrInt(MS)	N.m
Spring return torque:	TorqueSpInt(MS)	N.m
Inertia torque:	TorqueInInt(MS)	N.m
Resistant torque*:	TorqueReInt(MS)	N.m	or
External system
Friction torque:	TorqueFrExt(MS)	N.m
Spring return torque:	TorqueSpExt(MS)	N.m
Inertia torque:	TorqueInExt(MS)	N.m
Resistant torque *:	TorqueReExt(MS)	N.m	or

Reminder of the solved mechanical equation

The solved mechanical equation for rotating motion is:

where:

• J is the moment of inertia:

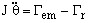
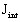• is the moment of inertia of the moving mechanical set itself (internal system)

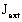• is the moment of inertia of the external load (external system)

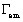• is the electromagnetic torque
• is the resistant torque:

• is the resistant torque acting on the moving mechanical set (internal system)
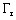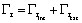
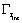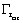• is the resistant torque acting on the external load (external system)

Important note

When there are symmetries or periodicities, the computed values of the torque correspond to the entire device.