RD-E: 0500 Beam Frame

A beam frame receives an impact from a mass having initial velocity.

A beam frame with clamped extremities receives an impact at its mid-point from a pointed mass having initial velocity. The material is subjected to the elasto-plastic law of Johnson-Cook. The model is meshed with beam elements. An infinite rigid wall with only one secondary node, including the impacted node, is subjected to the initial velocity. This example is considered a dynamic problem and the explicit solver is used.

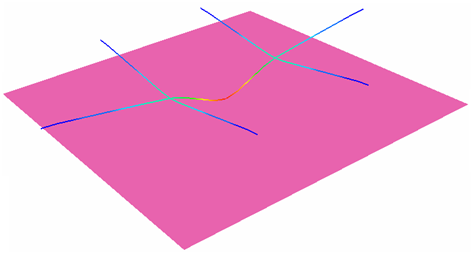
rad_ex_5_beam
Figure 1.

The explicit approach leads to finding a quasi-static equilibrium of the structure after impact.

Options and Keywords Used

The impacting mass is simulated using a sliding rigid plane wall (/RWALL) having an initial velocity of 10 ms-1and a mass of 3000 g. Only one secondary node exists: the node O to simulate a point impact.

Points A, F, F', D, E and E' are fully fixed.

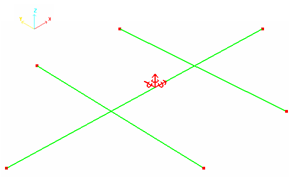
rad_ex_fig_5-3
Figure 2. Boundary Conditions

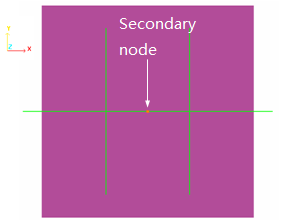
rad_ex_fig_5-4
Figure 3. Rigid Wall Type Infinite Plane

Input Files

Refer to Access the Model Files to download the required model file(s).

The model files used in this example are available in:

/radioss/example/05_Beam-frame

Model Description

The purpose of this example is to perform a static analysis using beam elements.

A pointed mass (3 kg) makes an impact at point O of a beam frame (see Figure 4 for the geometry) using a speed of 10 ms-1in the Z direction. The beams are made of steel and each beam section is square-shaped (each side being 6 mm long).

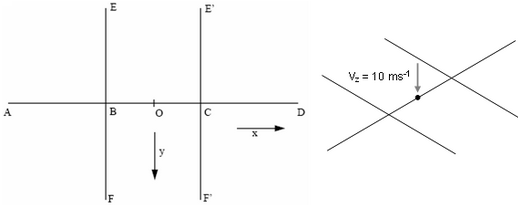
rad_ex_fig_5-1
Figure 4. Geometry of the Frame

Dimensions are: AB = BC = CD = BE = BF = E'C = CF' = 90 mm.

Points A, D, E, F, E', and F' are fixed.
Beam Properties
Cross section
36 mm2
Moments of inertia in Y and Z
108 mm4
Moments of inertia in X
216 mm4
The steel material used has the following properties:
Material Properties
Density
0.0078 [ g m m 3 ] MathType@MTEF@5@5@+= feaagKart1ev2aaatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9 vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaWaamWaaeaada WcaaqaaiaadEgaaeaacaWGTbGaamyBamaaCaaaleqabaGaaG4maaaa aaaakiaawUfacaGLDbaaaaa@3BBC@
Young's modulus
200 000 [ MPa ] MathType@MTEF@5@5@+= feaagKart1ev2aqatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqGqFfpeea0xe9vq=Jb9 vqpeea0xd9q8qiYRWxGi6xij=hbba9q8aq0=yq=He9q8qiLsFr0=vr 0=vr0db8meaabaqaciGacaGaaeqabaWaaeaaeaaakeaadaWadaqaai Gac2eacaGGqbGaaiyyaaGaay5waiaaw2faaaaa@3BE6@
Poisson's ratio
0.3
Yield stress
320 [ MPa ] MathType@MTEF@5@5@+= feaagKart1ev2aqatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqGqFfpeea0xe9vq=Jb9 vqpeea0xd9q8qiYRWxGi6xij=hbba9q8aq0=yq=He9q8qiLsFr0=vr 0=vr0db8meaabaqaciGacaGaaeqabaWaaeaaeaaakeaadaWadaqaai Gac2eacaGGqbGaaiyyaaGaay5waiaaw2faaaaa@3BE6@
Hardening parameter
134.65 [ MPa ] MathType@MTEF@5@5@+= feaagKart1ev2aqatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqGqFfpeea0xe9vq=Jb9 vqpeea0xd9q8qiYRWxGi6xij=hbba9q8aq0=yq=He9q8qiLsFr0=vr 0=vr0db8meaabaqaciGacaGaaeqabaWaaeaaeaaakeaadaWadaqaai Gac2eacaGGqbGaaiyyaaGaay5waiaaw2faaaaa@3BE6@
Hardening exponent
1.0

All other coefficients are set to default values. Plasticity is taken into account using LAW2 without failure.

Model Method

The mesh is a regular beam mesh, each beam being 9 mm long (total = 70 beams).

rad_ex_fig_5-2
Figure 5. Mesh of the Frame Showing the Position of the Nodes

Results

Curves and Animations

The main results refer to the time history of points B and O with regard to displacements and velocities.

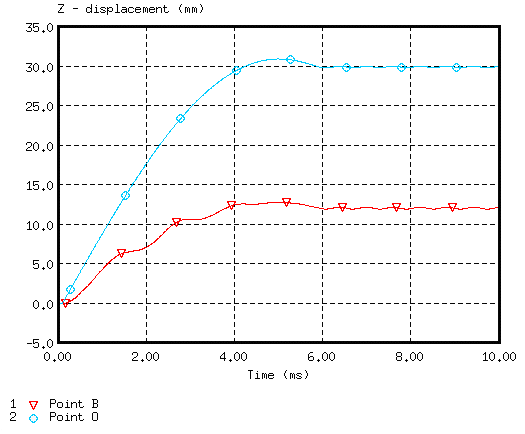
rad_ex_fig_5-5
Figure 6. Displacements of Points B and O

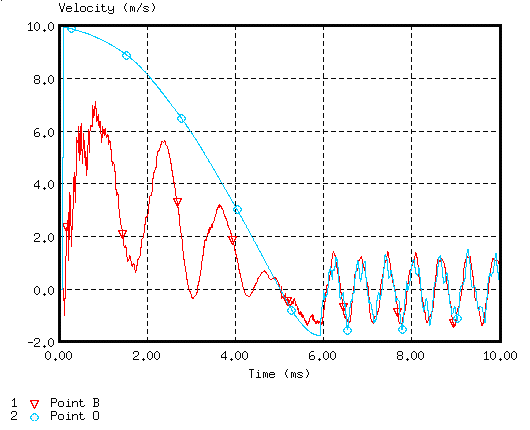
rad_ex_fig_5-6
Figure 7. Velocity of Points B and O (stabilization)

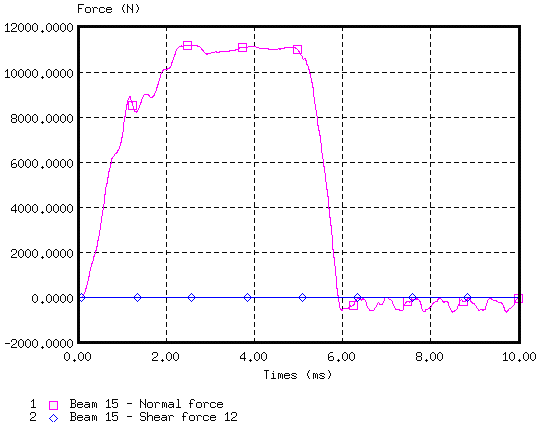
rad_ex_fig_5-7
Figure 8. Normal and Shear Force on Beam Element 15 (near to point O)

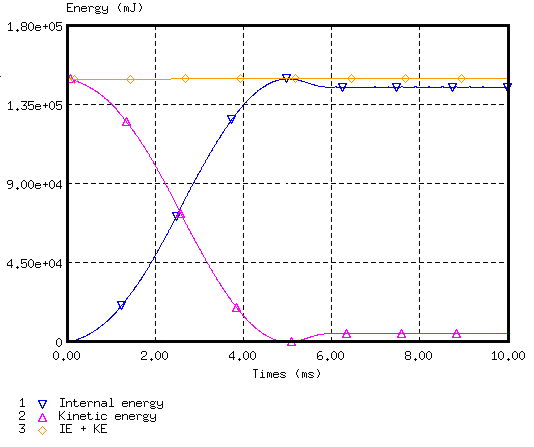
rad_ex_fig_5-8
Figure 9. Energy Assessment (stability reached at in 6 ms)

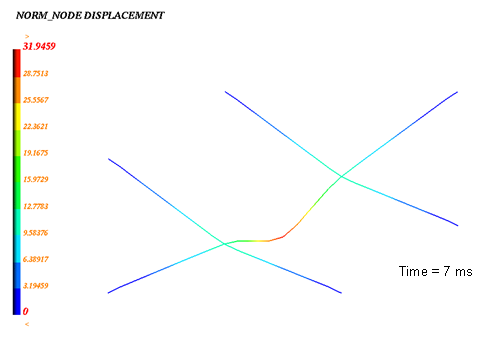
rad_ex_fig_5-9
Figure 10. Node Displacement (max. = 30.96 mm)

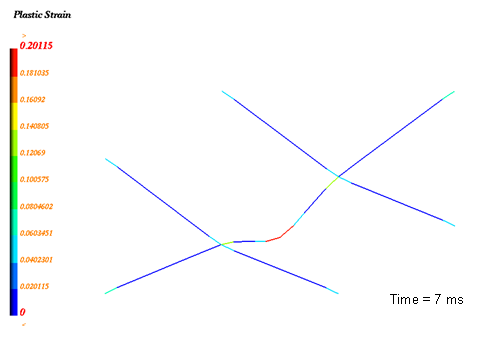
rad_ex_fig_5-10
Figure 11. Plastic Strain (max. = 20.1%)