Brittle Damage: Reinforced Concrete Material (LAW24)
The model is a continuum, plasticity-based, damage model for concrete. It assumes that the main two failure mechanisms are tensile cracking and compressive crushing of the concrete material.
The material law will enable to formulate the brittle elastic - plastic behavior of the reinforced concrete.
- E c
- Young's modulus
- Vc
- Poisson's ratio
- fc
- Uniaxial compressive strength
- fi/fc
- Tensile strength ratio
- fb/fc
- Biaxial strength ratio
- f2/fc
- Confined strength ratio
- s0/fc
- Confining stress ratio
- Second invariant of stress
- Mean stress
A schematic representation of the failure surface in the principal stress space is given in 図 2. The yield surface is derived from the failure envelope by introducing a scale factor . The meridian planes are presented in 図 3.
The steel directions are defined identically to material LAW14 by a TYPE6 property set. If a property set is not given in the element input data, r, s, are taken respectively as direction 1, 2, 3. For quad elements, direction 3 is taken as the direction.
- E
- Young's modulus
- Yield strength
- E t
- Tangent modulus
- Ratio of reinforcement in direction 1
- Ratio of reinforcement in direction 2
- Ratio of reinforcement in direction 3
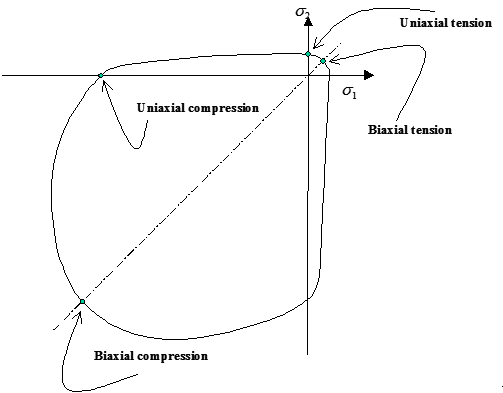
図 1. Failure Surface in Plane Stress
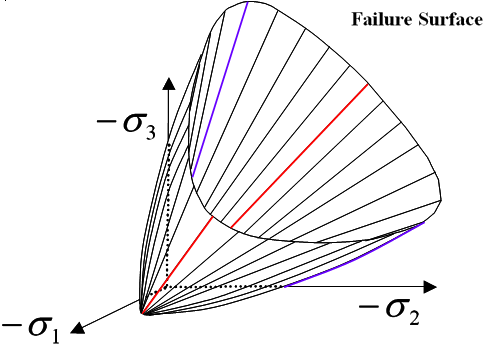
図 2. Failure Surface in Principal Stress Space
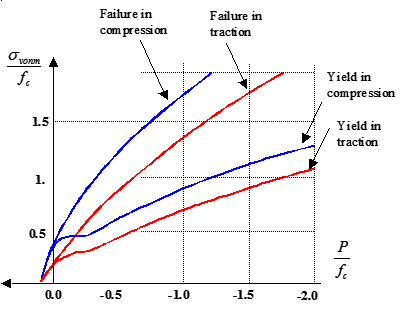
図 3. Meridians of Failure and Yield Surfaces