Johnson-Cook Plasticity Model (LAW2)

In this law the material behaves as linear elastic when the equivalent stress is lower than the yield stress.

For higher value of stress, the material behavior is plastic. This law is valid for brick, shell, truss and beam elements. The relation between describing stress during plastic deformation is given in a closed form:(1)

σ = (a + b ε_{p}^{n}) (1 + c 1 n \frac{\dot{ε}}{{\dot{ε}}_{0}}) (1 - T^{_{*} m})

Where,

$σ$: Flow stress (Elastic + Plastic Components)
$ε_{p}$: Plastic strain (True strain)
$a$: Yield stress
$b$: Hardening modulus
$n$: Hardening exponent
$c$: Strain rate coefficient
$\dot{ε}$: Strain rate
${\dot{ε}}_{0}$: Reference strain rate
$m$: Temperature exponent; $T^{*} = \frac{T - 298}{T_{m e l t} - 298}$
T_melt: Melting temperature in Kelvin degrees. The adiabatic conditions are assumed for temperature computation:(2) $T = T_{i} + \frac{E_{int}}{ρ C_{ρ} (V o l u m e)}$

Where,

$ρ C_{p}$: Specific heat per unit of volume
$T_{i}$: Initial temperature (in degrees Kelvin)
$E_{int}$: Internal energy

Two optional additional inputs are:

$σ_{\max 0}$: Maximum flow stress
$ε_{\max}$: Plastic strain at rupture

図 1 shows a typical stress-strain curve in the plastic region. When the maximum stress is reached during computation, the stress remains constant and material undergoes deformation until the maximum plastic strain. Element rupture occurs if the plastic strain is larger than

ε_{\max}

. If the element is a shell, the ruptured element is deleted. If the element is a solid element, the ruptured element has its deviatoric stress tensor permanently set to zero, but the element is not deleted. Therefore, the material rupture is modeled without any damage effect.

C_hard in this material law is same like in /MAT/LAW44. For more details on C_hard, refer to Cowper-Symonds Plasticity Model (LAW44).

Strain Rate Definition

Regarding to the plastification method used, the strain rate expression is different. If the progressive plastification method is used (that is, integration points through the thickness for thin-walled structured), the strain rate is:(3)

\frac{d ε}{d t} = \max (\frac{d ε_{x}}{d t}, \frac{d ε_{y}}{d t}, 2 \frac{d}{d t} (ε_{x y}))

(4)

ε ​ {}_{x y}= \frac{1}{2} γ ​ {}_{x y}

With global plastification method:(5)

\frac{d ε}{d t} = (\frac{d E_{i} / d t}{σ_{V M}})

Where, $E_{i}$ is the internal energy.

For solid elements, the maximum value of the strain rate components is used:(6)

\dot{ε} = \max ({\dot{ε}}_{x}, ​ {\dot{ε}}_{y}, {\dot{ε}}_{z}, 2 {\dot{ε}}_{x y}, 2 {\dot{ε}}_{y z}, 2 {\dot{ε}}_{x z})

Strain Rate Filtering

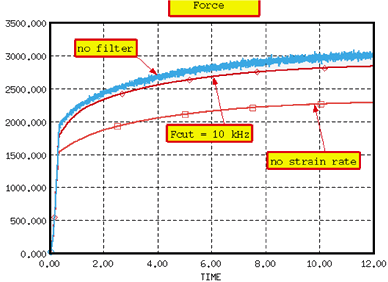
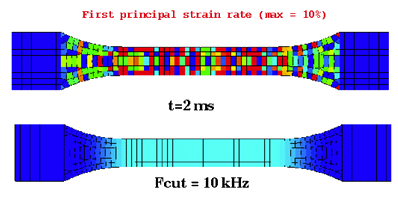
The strain rates exhibit very high frequency vibrations which are not physical. The strain rate filtering option will enable to damp those oscillations and; therefore obtain more physical strain rate values.

If there is no strain rate filtering, the equivalent strain rate is the maximum value of the strain rate components:(7)

{\dot{ε}}_{e q} = \max ({\dot{ε}}_{x^{'}} {\dot{ε}}_{y^{'}} {\dot{ε}}_{z^{'}} 2 {\dot{ε}}_{x y^{'}} 2 {\dot{ε}}_{y z^{'}} 2 {\dot{ε}}_{X Z})

For thin-walled structures, the equivalent strain is computed by the following approach. If ε is the main component of strain tensor, the kinematic assumptions of thin-walled structures allows to decompose the in-plane strain into membrane and flexural deformations:(8)

ε = K Z = ε_{m}

Then, the expression of internal energy can by written as:(9)

E_{i} = \int_{- \frac{t}{2}}^{\frac{t}{2}} σ ε d z = \int_{- \frac{t}{2}}^{\frac{t}{2}} E ε^{2} d z = \int_{- \frac{t}{2}}^{\frac{t}{2}} E {(κ z + ε_{m})}^{2} d z

Therefore:(10)

E_{i} = \int_{- \frac{t}{2}}^{\frac{t}{2}} E (κ^{2} z^{2} + ε_{m}^{2} + 2 κ ε_{m} z) d z = E {(\frac{1}{3} κ^{2} z^{3} + ε_{m}^{2} z + κ ε_{m}^{} z^{2})}_{- \frac{t}{2}}^{\frac{t}{2}}

The expression can be simplified to:(11)

E_{i} = E [\frac{1}{12} κ^{2} t^{3} + ε_{m}^{2} t] = E ε_{e q}^{2} ​ t

(12)

ε_{e q} = \sqrt{\frac{1}{12} κ^{2} t^{2} + ε_{m}^{2}}

The expression of the strain rate is derived from 式 8:(13)

\dot{ε} = \dot{K} Z + {\dot{ε}}_{m}

Admitting the assumption that the strain rate is proportional to the strain, that is:(14)

{\dot{ε}}_{m} = α ε_{m}

(15)

\dot{K} = α K

Therefore:(16)

\dot{ε} = α ε

Referring to 式 12, it can be seen that an equivalent strain rate can be defined using a similar expression to the equivalent strain:(17)

{\dot{ε}}_{e q} = α ε_{e q}

(18)

{\dot{ε}}_{e q} = \sqrt{\frac{1}{12} κ^{2} t^{2} + {\dot{ε}}_{m}^{2}}

For solid elements, the strain rate is computed using the maximum element stretch:(19)

{\dot{ε}}_{e q} = {\dot{λ}}_{\max}

The strain rate at integration point,

i

in /ANIM/TENS/EPSDOT/i

(1 < i < n)

is calculated by:(20)

{\dot{ε}}_{i} = {\dot{ε}}_{m} - \frac{1}{2} (\frac{(2 i - 1)}{n} - 1) t {\dot{ε}}_{b}

Where,

${\dot{ε}}_{m}$: Membrane strain rate /ANIM/TENS/EPSDOT/MEMB
${\dot{ε}}_{b}$: Bending strain rate /ANIM/TENS/EPSDOT/BEND.

The strain rate in upper and lower layers is computed by:(21)

{\dot{ε}}_{u} = ε_{m} + \frac{1}{2} t {\dot{ε}}_{b}

/ANIM/TENS/EPSDOT/UPPER

(22)

{\dot{ε}}_{1} = {\dot{ε}}_{m} - \frac{1}{2} t {\dot{ε}}_{b}

/ANIM/TENS/EPSDOT/LOWER

The strain rate is filtered by using:(23)

{\dot{ε}}_{f} (t) = a \dot{ε} (t) + (1 - a) {\dot{ε}}_{f} (t - 1)

Where,

$a=2 π {dt F}_{c u t}$
$dt$: Time interval
F_cut: Cutting frequency
${\dot{ε}}_{f}$: Filtered strain rate

Strain Rate Filtering Example

An example of material characterization for a simple tensile test RD-E：1100 引張試験 is given in Radioss Example Guide. For the same example, a strain rate filtering allows to remove high frequency vibrations and obtain smoothed the results. This is shown in 図 2 and 図 3 where the cut frequency F_cut = 10 KHz is used.