RD-E: 0601 Fluid Structure Coupling
Sloshing inside a fuel tank by simulating the fluid structure coupling. The tank deformation is achieved by applying an imposed velocity on the left corners. Water and air inside the tank are modeled with the ALE formulation. The tank container is described using a Lagrangian formulation.
A numerical simulation of fluid-structure coupling is performed on sloshing inside a deformable fuel tank. This example uses the ALE (Arbitrary Lagrangian Eulerian) formulation and the hydrodynamic bi-material law (/MAT/LAW37) to model interaction between water, air and the tank container.
Options and Keywords Used
- Fluid structure coupling simulation, and ALE formulation
- Shell and brick elements
- Hydrodynamic and bi-phase liquid gas material (/MAT/LAW37 (BIPHAS))
- ALE boundary conditions (/ALE/BCS)
- J. Donea Grid Formulation (/ALE/GRID/DONEA)
- Boundary conditions (/BCS)
- Gravity (/GRAV)
- Imposed velocity (/IMPVEL)
- ALE material formulation (/ALE/MAT)
| Velocity (ms-1) | 0 | 5 | 0 | 0 |
| Time (ms) | 0 | 12 | 12.01 | 50 |
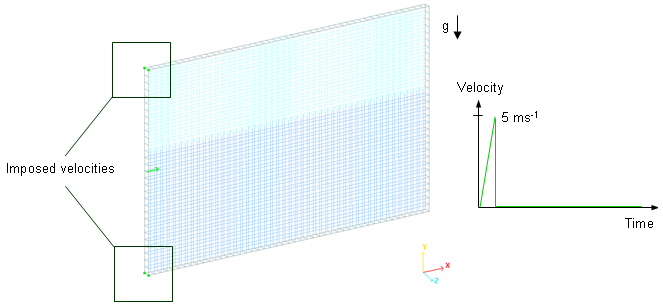
Figure 1. Kinematic Condition: Imposed Velocities
- Material velocity
- Grid velocity
All nodes, except those on the border have grid (/ALE/BCS) and material (/BCS) velocities fixed in the Z-direction. The nodes on the border only have a material velocity (/BCS) fixed in the Z-direction.
The /ALE/GRID/DONEA option activates the J. Donea grid formulation to compute the grid velocity. See the Radioss Theory Manual for further explanations about this option.
Input Files
Refer to Access the Model Files to download the required model file(s).
The model files used in this example include:
TANK_*.rad
Model Description
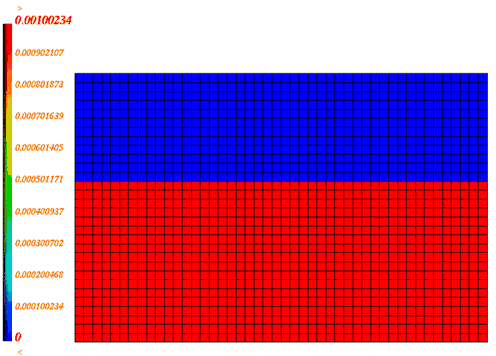
A rectangular tank made of steel is partially filled with water, the remainder being supplemented by air. The initial distribution pressure is known and supposed homogeneous. The tank container dimensions are 460 mm x 300 mm x 10 mm, with thickness being at 2 mm.
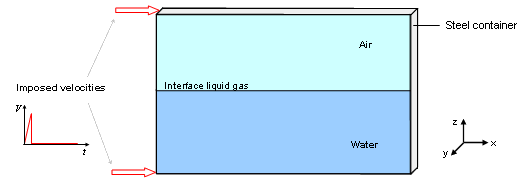
Figure 2. Problem Description
- Material Properties
- Density
- 0.0078
- Young's modulus
- 210000
- Poisson's ratio
- 0.29
- Yield stress
- 180
- Hardening parameter
- 450
- Hardening exponent
- 0.5
The material air-water bi-phase is described in the hydrodynamic bi-material liquid-gas law (/MAT/LAW37). Material LAW37 is specifically designed to model bi-material liquid gas.
- Viscosity
- Liquid EOS
- Gas EOS
The equilibrium is defined by:
- Deviatoric stress tensor
- Deviatoric strain tensor
- Material Parameters - For Liquid
- Liquid reference density,
- 0.001
- Liquid bulk modulus,
- 2089 N/mm2
- Initial mass fraction liquid proportion,
- 100%
- Shear kinematic viscosity ,
- 0.001 mm2/ms
- Material Parameters - For Gas
- Gas reference density,
- 1.22x10-6
- Shear kinematic viscosity ,
- 0.00143 mm2/ms
- Constant perfect gas,
- 1.4
- Initial pressure reference gas,
- 0.1 N/mm2
- Properties
- Quadratic bulk viscosity/linear bulk viscosity
- 10-20
- Hourglass bulk coefficient
- 10-5
Model Method
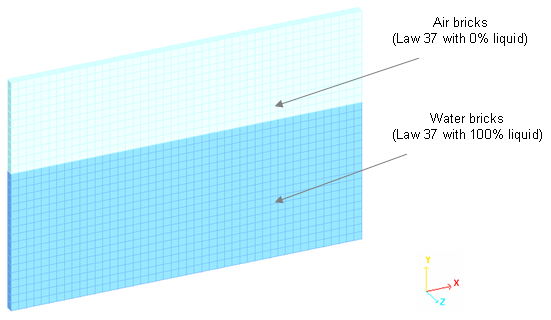
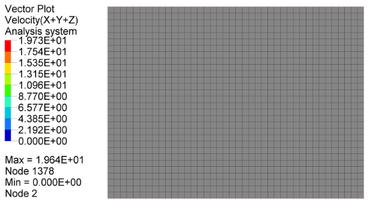
Figure 3. Air and Water Mesh (ALE brick elements)
Figure 4. Tank Container Mesh (shell elements)
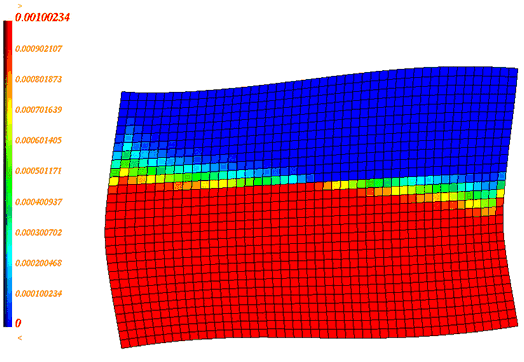
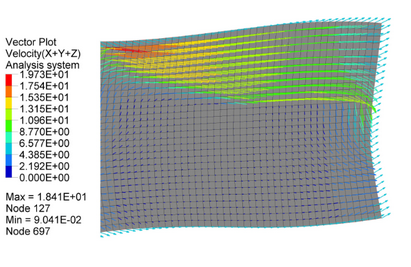
Using the ALE formulation, the brick mesh is only deformed by tank deformation the water flowing through the mesh. The Lagrangian shell nodes still coincide with the material points and the elements deform with the material: this is known as a Lagrangian mesh. For the ALE mesh, nodes on the boundaries are fixed in order to remain on the border, while the interior nodes are moved.
Results
Curves and Animations
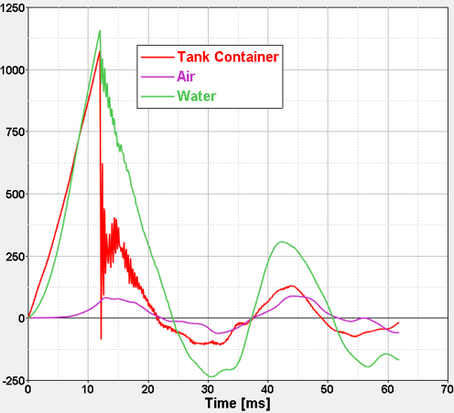
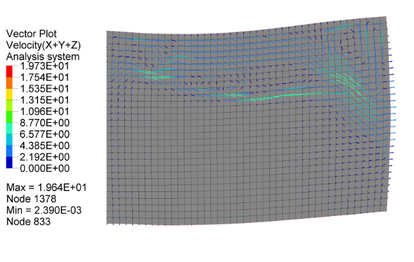
Figure 5. X-Momentum Variation for Each Part
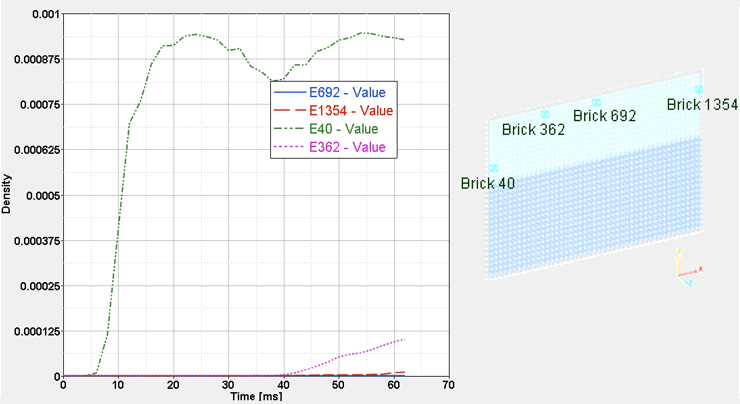
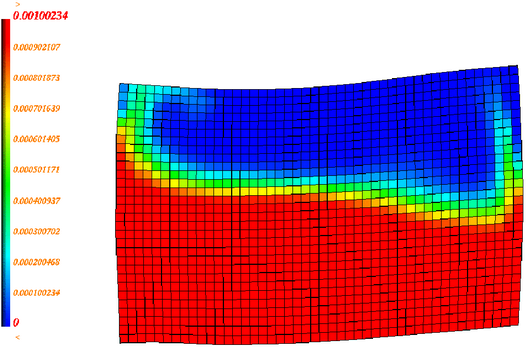
Figure 6. Density Attached to the Various Brick Elements
| Density
|
|
| Velocity
|
| Density
|
|
| Velocity
|
| Density
|
|
| Velocity
|